Calculus is a branch of mathematics that deals with rates of change and the accumulation of quantities. Whether you’re looking to understand the basics for academic purposes, career development, or personal enrichment, having a solid grasp of essential calculus concepts can greatly enhance your mathematical skills. This guide provides a comprehensive overview of fundamental principles, designed for beginners.
1. Understanding Calculus
Calculus is divided mainly into two branches: Differential Calculus and Integral Calculus. Differential Calculus focuses on the concept of the derivative, which measures how a function changes as its input changes. Integral Calculus, on the other hand, deals with the accumulation of quantities, represented by the integral.
2. Limits and Continuity
Limits are a foundational concept in calculus. They describe the value that a function approaches as the input approaches a particular point. Understanding limits is crucial for defining both derivatives and integrals.
Continuity refers to whether a function is smooth and unbroken. A function is continuous at a point if the limit of the function exists and is equal to the function’s value at that point. In simpler terms, a function is continuous if you can draw it without lifting your pencil.
3. Derivatives
The derivative measures the rate of change of a function. It can be thought of as the slope of the tangent line to the graph of the function at a given point. To find the derivative, you often use rules and formulas such as the power rule, product rule, quotient rule, and chain rule.
Power Rule: For any function of the form f(x)=xnf(x) = x^nf(x)=xn, the derivative is f′(x)=nxn−1f'(x) = nx^{n-1}f′(x)=nxn−1.
Product Rule: For functions u(x)u(x)u(x) and v(x)v(x)v(x), the derivative of their product is (uv)′=u′v+uv′(uv)’ = u’v + uv’(uv)′=u′v+uv′.
Quotient Rule: For functions u(x)u(x)u(x) and v(x)v(x)v(x), the derivative of their quotient is (uv)′=u′v−uv′v2\left(\frac{u}{v}\right)’ = \frac{u’v – uv’}{v^2}(vu)′=v2u′v−uv′.
Chain Rule: To differentiate a composite function f(g(x))f(g(x))f(g(x)), use (f(g(x)))′=f′(g(x))⋅g′(x)(f(g(x)))’ = f'(g(x)) \cdot g'(x)(f(g(x)))′=f′(g(x))⋅g′(x).
4. Integrals
Integrals represent the accumulation of quantities and can be thought of as the area under a curve. The process of finding an integral is called integration. There are two main types: definite integrals and indefinite integrals.
Definite Integral: Calculates the total accumulation from one point to another and is written as ∫abf(x) dx\int_a^b f(x) \, dx∫abf(x)dx.
Indefinite Integral: Represents a family of functions and is written as ∫f(x) dx\int f(x) \, dx∫f(x)dx, including a constant of integration CCC.
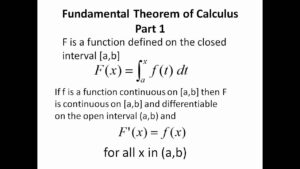
5. Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus links the concept of the derivative with the concept of the integral. It has two main parts:
- First Part: If F(x)F(x)F(x) is an antiderivative of f(x)f(x)f(x), then ∫abf(x) dx=F(b)−F(a)\int_a^b f(x) \, dx = F(b) – F(a)∫abf(x)dx=F(b)−F(a).
- Second Part: If fff is continuous on [a,b][a, b][a,b], then F(x)=∫axf(t) dtF(x) = \int_a^x f(t) \, dtF(x)=∫axf(t)dt is an antiderivative of fff.
6. Applications of Calculus
Calculus has a wide range of applications across various fields, including:
- Physics: Describing motion, forces, and energy.
- Engineering: Analyzing systems and designing structures.
- Economics: Modeling and optimizing functions related to costs and revenues.
- Biology: Understanding population dynamics and growth rates.
7. Common Challenges and Tips
Common Challenges: Many beginners struggle with understanding the abstract nature of calculus concepts and applying them to solve problems.
Tips:
- Practice Regularly: Regular practice helps in reinforcing concepts and solving different types of problems.
- Visualize: Use graphs and diagrams to understand the behavior of functions and their derivatives or integrals.
- Seek Help: Don’t hesitate to ask for help from teachers, tutors, or online resources when you’re stuck.
8. Conclusion
Mastering essential calculus concepts opens the door to understanding more complex mathematical theories and applications. By grasping the basics of limits, derivatives, and integrals, and how they interrelate through the Fundamental Theorem of Calculus, you lay a strong foundation for further study.



