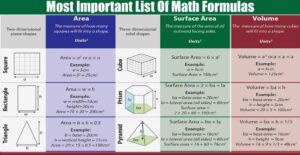
Geometry is a fundamental branch of mathematics that deals with shapes, sizes, and the properties of space. Mastery of key geometry formulas is crucial for students, as these formulas provide the tools necessary for solving problems related to various geometric figures. In this article, we’ll explore the top 10 essential geometry formulas that every student should know. These formulas will not only help in academic pursuits but also in real-world applications.
1. Area of a Triangle
The area of a triangle can be calculated using the formula: Area=12×base×height\text{Area} = \frac{1}{2} \times \text{base} \times \text{height} where the base is any one side of the triangle, and the height is the perpendicular distance from the base to the opposite vertex. This formula is fundamental for solving problems involving triangles in various contexts.
2. Area of a Rectangle
To find the area of a rectangle, use the formula: Area=length×width\text{Area} = \text{length} \times \text{width} where length and width are the dimensions of the rectangle. This formula is simple yet crucial for calculating the space inside rectangular shapes, from room dimensions to surface areas.
3. Area of a Circle
The area of a circle is given by: Area=π×r2\text{Area} = \pi \times r^2 where rr is the radius of the circle. This formula is essential for problems involving circular objects, such as calculating the size of a circular garden or the surface area of a round table.
4. Circumference of a Circle
To find the circumference of a circle, use: Circumference=2×π×r\text{Circumference} = 2 \times \pi \times r where rr is the radius of the circle. The circumference is the distance around the circle and is important for tasks such as determining the length of fencing needed for a circular area.
5. Area of a Parallelogram
The area of a parallelogram can be calculated using: Area=base×height\text{Area} = \text{base} \times \text{height} where the base is one of the sides of the parallelogram and the height is the perpendicular distance between the two parallel sides. This formula is useful for finding areas of shapes with parallel sides.
6. Area of a Trapezoid
The formula for the area of a trapezoid is: Area=12×(base1+base2)×height\text{Area} = \frac{1}{2} \times (\text{base}_1 + \text{base}_2) \times \text{height} where base1\text{base}_1 and base2\text{base}_2 are the lengths of the two parallel sides, and the height is the perpendicular distance between these bases. This formula is commonly used in engineering and design fields.
7. Pythagorean Theorem
In a right triangle, the Pythagorean Theorem states: a2+b2=c2a^2 + b^2 = c^2 where aa and bb are the lengths of the two legs, and cc is the length of the hypotenuse. This theorem is fundamental in geometry and trigonometry, used to find distances and solve problems involving right triangles.
8. Volume of a Rectangular Prism
The volume of a rectangular prism is calculated by: Volume=length×width×height\text{Volume} = \text{length} \times \text{width} \times \text{height} This formula is vital for determining the capacity of three-dimensional rectangular objects, such as boxes or rooms.
9. Volume of a Cylinder
To find the volume of a cylinder, use: Volume=π×r2×h\text{Volume} = \pi \times r^2 \times h where rr is the radius of the base and hh is the height of the cylinder. This formula is useful for calculating the capacity of cylindrical objects, such as pipes or tanks.
10. Volume of a Sphere
The volume of a sphere is given by: Volume=43×π×r3\text{Volume} = \frac{4}{3} \times \pi \times r^3 where rr is the radius of the sphere. This formula is essential for determining the volume of spherical objects, such as balloons or planets.
Conclusion
Mastering these essential geometry formulas is crucial for students at all levels. They provide a solid foundation for understanding and solving geometric problems, both in academic settings and real-life applications. By familiarizing yourself with these formulas, you’ll be better equipped to tackle various geometry challenges and improve your overall mathematical skills.
